Measuring temperatures and thermal expansion

Key questions

What different temperature scales are there, and what are they based on?
How is temperature measured?
How can thermal expansion be modelled mathematically?
In which situations is thermal expansion important?

Temperature as a quantity

Temperature is a familiar quantity. Temperatures on Earth vary by season and usually range by fifty degrees Celsius, depending on where you are. In everyday life, temperatures are usually a little above zero degrees Celsius, but some higher temperatures can be found in our environment. Water boils at one hundred degrees Celsius, and we cook food in ovens at up to 300 degrees. Industry uses even higher temperatures, for example, to melt metal. Conversely, humans are able to create significantly lower temperatures than those that occur naturally on Earth. Liquid helium cools objects to –269 degrees Celsius. Temperatures in space fluctuate even more than those created by humans: The temperature at the Sun’s core is around 15 million degrees Celsius, while empty space is –270 degrees Celsius.

Temperatures on Earth range from searing heat in deserts to plummeting sub-zero temperatures at the poles. On the left is the Umm Al Ma lake in the Awbari desert in Libya, and on the right is a Siberian forest in winter

Humans are capable of sensing approximate temperature differences in objects. This sense is based on differences in temperature balancing themselves out. A person senses whether an object is hotter or colder than their skin. Nevertheless, even at the same temperatures, different materials can feel colder or hotter. For example, the wooden part of a desk feels warmer than the metal legs. This is because materials conduct heat differently. This is why measuring temperature by sense is imprecise.

A temperature can be assigned a more precise scale without knowing what the exact temperature is. In order to create a scale, we need two points that are assigned to certain temperatures. The Celsius scale presented by Swede Anders Celsius (1701–1744) was originally determined in the 18th century as follows:

the melting point of ice was determined to be zero degrees and
the boiling point of water was one hundred degrees.

Anders Celsius (Wikipedia)

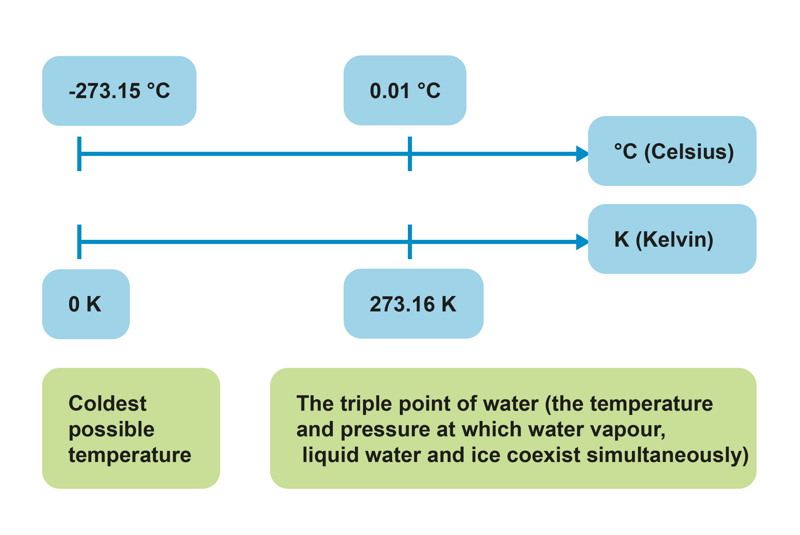
Temperature is usually denoted using the symbol T and it is measured in multiple units. Along with the Celsius scale, the sciences also make use of the Kelvin scale presented by the Irish-British engineer and physicist Lord Kelvin (1824–1907). The Kelvin scale is built around the lowest possible temperature and the temperature of the triple point of water. There are no negative values on the Kelvin scale, so its lowest possible temperature, or absolute zero, is 0 K.

Lord Kelvin (Wikipedia)

The temperature 0 K is equal to –273.15°C. A change in temperature has the same value in Celsius and in Kelvin; so the change in one Kelvin is the same as the change in one degree Celsius.

1. Humans are capable of sensing approximate temperature differences in objects.

True
False

2. A measurement scale can be determined for a temperature by choosing two points that are assigned to certain temperatures.

True
False

3. The Celsius scale is based on the lowest possible temperature and the temperature of the triple point of water.

True
False

4. A change in temperature has the same value in Celsius and in Kelvin.

True
False

Lisää aiheesta

Measuring temperature

Many technically different devices have been developed for measuring temperature. The majority of them are based on the observation of materials expanding as they are heated. This is known as thermal expansion. Liquid-in-glass thermometers contain a liquid that expands, and the change in the height of the column of liquid in the tube is used to measure the temperature. The video below shows an example of such a thermometer. A digital thermometer, on the other hand, contains an electronic component with measuring properties that depend on the temperature.

Different kinds of thermometers

A Galileo thermometer contains different-coloured containers of liquid with different densities. The colourless liquid expands as it warms up and its density reduces, so the containers of liquid sink to the bottom one by one as the temperature rises.

The liquid expands as it warms up, so the height of the liquid column in the thermometer increases.

Digital thermometers usually contain an element whose opposition to the flow of electric current (resistance) changes with temperature. The thermometer measures resistance and then displays a temperature based on the resistance.

A thermometer with a dial contains a spring coil made from two different metals.

Lisää aiheesta

Linear thermal expansion

Let’s examine thermal expansion in a metal bar with a length of one metre. To the eye, the bar does not significantly lengthen, which is typical for objects that we encounter in our daily lives. The picture below shows the equipment used.

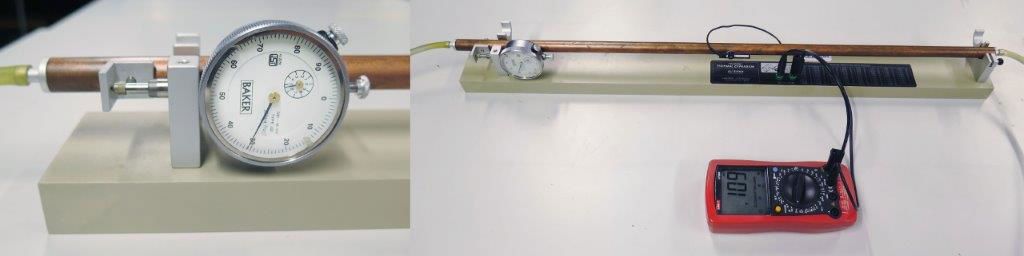
The meter to the left-hand side of the bar measures changes in the length of the bar to a precision of 0.01 mm. The red meter is a multimeter that measures resistance. If we know the resistance of a component connected to the pipe at different temperatures, we can check the temperature table to find the temperature that corresponds to the resistance measured. The temperature of the pipe is measured by running water through it at different temperatures.

The measurement results are shown on a graph, with the temperature in degrees Celsius along the horizontal axis and the change in the length of the bar in millimetres along the vertical axis.

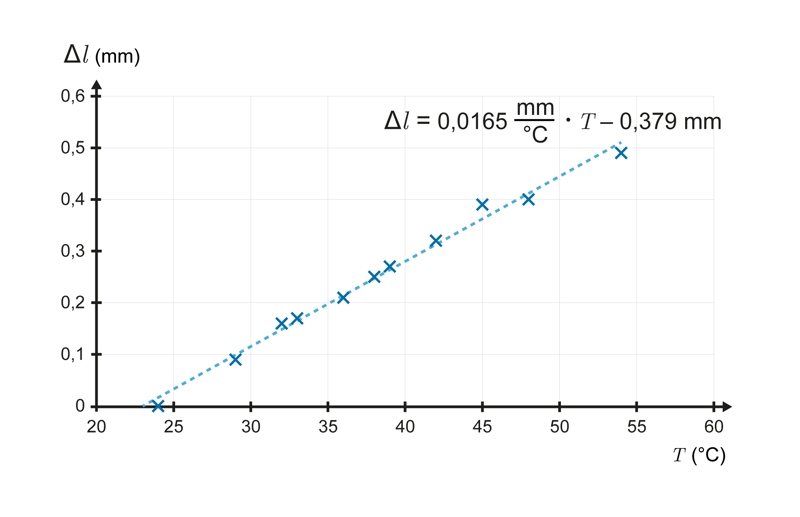
Measurement results

Linear_thermal_expansion.ods (LibreCalc)

Linear_thermal_expansion.cmbl (Logger Pro)

Linear_thermal_expansion.cap (Capstone)

The measurement points form a line with an equation of $Δ l = 0.0165 m m /^{\circ} C \cdot T - 0.379 m m$ . Linearity tells us that the bar lengthens evenly as the temperature rises. The gradient of the line is calculated by dividing the change on the vertical axis by the change on the horizontal axis. It has a size of 0.0165 and the unit is mm/°C. The size of the gradient tells us that the length of the bar has increased by 0.0165 millimetres as the temperature rose by one degree Celsius. Correspondingly, as the temperature decreases, the bar shortens by 0.0165 millimetres for each Celsius degree.

If identical bars are placed one behind the other, each bar will lengthen by 0.0165 millimetres per degree Celsius and the length of the bars will increase by a total of the number of bars multiplied by 0.0165 millimetres. Therefore, when heated, a bar with length $l$ will lengthen

$l \cdot 0.0165 \frac{mm}{m °C} = l \cdot 0.000 0165 \frac{1}{°C} .$

The final length of the bar is obtained by adding the change in length caused by the temperature to the original length.

Linear thermal expansion

The length of the object $l$ after a change in temperature $Δ T$ is

$l = l_{0} + l_{0} α Δ T,$

where $l_{0}$ is the length before the change in temperature and $α$ is the empirically determined coefficient of linear thermal expansion.

The coefficient of linear thermal expansion is individual to each material. In the case of the bar we examined, the coefficient was observed to be 0.0000165 per 1/°C. Linear thermal expansion coefficients for different materials are listed in the table book. The thermal expansion coefficient of copper is 0.0000168 per 1/°C, so the bar we examined could have been a copper bar.

Thermal expansion coefficients for various materials (Wikipedia)

Lisää aiheesta

Thermal expansion in nature

Thermal expansion is observed in many other situations besides temperature measurement. Thermal expansion is often a detrimental process and we strive to minimise its effects. For example, a bridge made from steel beams or concrete expands as it warms up and contracts as it cools. Flexible joints must be built for the bridge to make sure that the structures do not break due to thermal expansion. The same applies to other large structures.

Flexible joints or seams are also required when combining different materials if one of the materials expands more than the other. For example, an aluminium window frame expands more than the glass pane inside it. An inflexible seam would cause problems.

Water is a good example of exceptional thermal expansion. As the temperature of water rises from four degrees upwards, water expands. However, it also expands as the temperature falls from four degrees towards the freezing point. Therefore, water takes up the least space at four degrees, when its density is highest. This is of key importance to the planet’s waters. If water were at its densest at zero degrees, water at its freezing point would sink to the bottom of the waterway and freezing would begin from the bottom. This would make it difficult for plants and animals in them to survive.

Flexible joints are built for bridges to prevent the structures from becoming damaged as a result of thermal expansion.

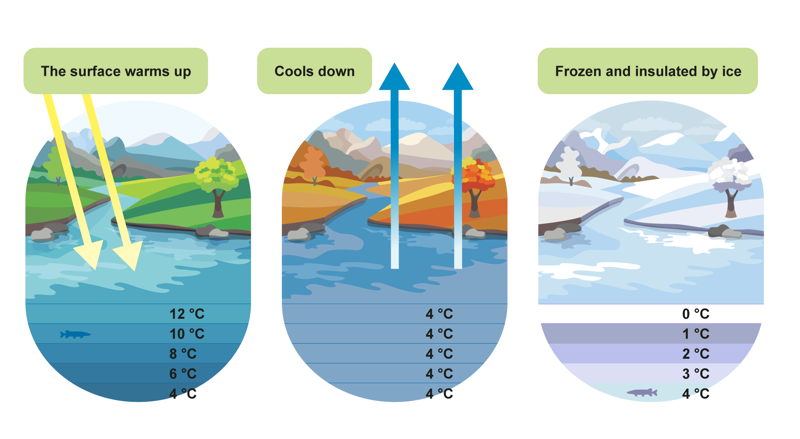
The images show that in summer, water starts to warm up from the surface. The warm water remains on the surface and the colder, denser water sinks to the bottom. In autumn, the water freezes from the surface, and the water cooling to four degrees water sinks towards the bottom. If there is any warm water left at depth, it will rise to the surface. Once the entire waterway has cooled to four degrees, the surface water will cool further. There is less water that cools below four degrees than water that is at four degrees, so it remains on the surface and before long, it freezes. This top layer of ice acts as an insulator and slows the cooling process for the rest of the water.

Thermal expansion can also be observed in issues that affect the entire planet. As the climate heats up, sea temperatures rise. In addition to glacial melting, the thermal heat expansion of seawater also causes sea levels to rise. The scale of the impact of thermal expansion has not yet been precisely ascertained, but many tropical islands and coastal cities are in danger of being submerged.

The rise in sea levels as a result of global warming threatens low-lying tropical islands. A lagoon and Mount Otemanu on the island of Bora Bora in Tahiti.

Lisää aiheesta

Volumetric thermal expansion

Objects expand in every direction when heated. A bar has much more length than depth or width, and that’s why we mostly observe its expansion in length. If the object resembles a cube more than a wire, the thermal expansion will be the same in every direction. In such cases, changes in length in all directions must be taken into account.

Liquids take on the shape of their container. If the thermal expansion of a liquid is stronger than that of the container, we will observe thermal expansion as a rise in the surface of the liquid. A full and closed container of liquid may break if the liquid expands more strongly than the material of the container. Unless the thermal expansion of the container is known to be trivial, it must be taken into account when investigating the thermal expansion of a liquid.

balance themselves out
kelvin
0 K
expand
degrees Celsius
273 K

1. Temperature is a quantity with a base unit of and another common unit is the .

2. The lowest possible temperature is .

3. The melting point of water is around .

4. Temperature differences naturally.

5. Most materials as they are heated.

Volumetric thermal expansion

The volume of the material $V$ after the change in temperature $Δ T$ is

$V = V_{0} + V_{0} γ Δ T,$

where $V_{0}$ is the volume before the change in temperature and $γ$ is the empirically determined coefficient of volumetric thermal expansion.

Gases expand even more strongly than liquids when they are heated. However, the situation for gases is more complex than for solids and liquids, as gases can be compressed. Changes in the volume of gases will be examined in the section State of gases and changes of state

Lisää aiheesta

Examples

Example 1

The lowest temperature overnight was 8.3°C and the highest temperature in the daytime was 23.1°C. Give these temperatures and the change in temperature in kelvins.
Helium changes from a gas into a liquid at a temperature of 4.22 K. Give this temperature in degrees Celsius.

Solution to example 1

Solution to section a)

We can convert degrees Celsius into kelvins by adding 273.15. Therefore

$8.3 °C = (8.3 + 273.15) K = 281.45 K \approx 281.5 K$

$23.1 °C = (23.1 + 273.15) K = 296.25 K \approx 296.3 K$

The change in temperature has the same value in kelvins and in degrees Celsius:

$23.1 °C - 8.3 °C = 14.8 °C$

$296.3 K - 281.5 K = 14.8 K$

The change in temperature always has the same value in kelvins and in degrees Celsius.

The temperatures are 281.5 K and 296.3 K. The change in temperature is 14.8 K.

Solution to section b)

We can convert kelvins into degrees Celsius by subtracting 273.15. Therefore

$4.22 K = (4.22 - 273.15) °C = - 268.93 °C$

Helium changes from a gas into a liquid at a temperature of –268.93°C.

Example 2

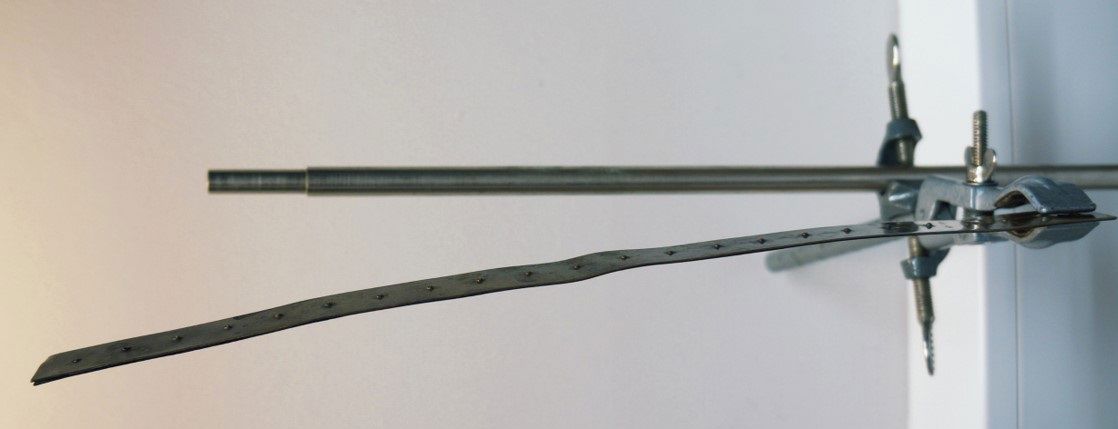
Two long, thin strips of equal length have been made from different metals and are stapled together at several points. The metals have different coefficients of linear thermal expansion. What will happen to the double metal strip when it is heated?

A heated double metal strip attached to a stand.

Solution to example 2

The lengths of the strips increase as they are heated. One stretches more than the other, however, which makes the double metal strip curve. A dial thermometer uses a coiled double metal strip to which a hand is added.

Example 3

The length of an iron bar at 18°C is 1265.0 mm.

The bar is heated to 315°C. What is the new length of the bar?
How much do we need to heat the bar in order for it to increase in length by 5.0 mm?

Solution to example 3

Solution to section a)

The length of the bar increases steadily as it is heated. Lengthening takes place according to

$l = l_{0} + l_{0} α Δ T,$ where

$l$ is the new length
$l_{0}$ is the original length
$α$ is the coefficient of linear thermal expansion and
$Δ T$ is the change in temperature.

Now
$l_{0} = 1 265.0 mm$
$α = 11.8 \cdot 10^{- 6} 1 / °C$
$Δ T = 315 °C - 18 °C = 297 °C$

We get

$\begin{aligned} l & = 1 265.0 mm + 1 265.0 mm \cdot 11.8 \cdot 10^{- 6} 1 / °C \cdot 297 °C \\ = 1 269.43 \dots mm \approx 1 269.4 mm \end{aligned}$

The new length of the bar is 1269.4 millimetres.

Solution to section b)

Change in length in thermal expansion

$\begin{aligned} Δ l & = l_{0} α Δ T \\ Δ T & = \frac{Δ l}{l_{0} α} \end{aligned}$

Now
$Δ l = 5.0 mm$
$l_{0} = 1 265.0 mm$
$α = 11.8 \cdot 10^{- 6} 1 / °C$

$Δ T = 334.963^{\circ} C \approx 335^{\circ} C$

The bar must be heated to approx. 335°C.

Example 4

A metal bar has a length of 826.0 mm at 23°C and of 830.7 mm at 269°C. What material is the bar made of?

Solution to example 4

The change in the length of the bar depends on the material the bar is made of. Lengthening takes place according to

$l = l_{0} + l_{0} α Δ T,$ where

$l$ is the new length
$l_{0}$ is the original length
$α$ is the coefficient of linear thermal expansion and
$Δ T$ is the change in temperature.

The coefficient of linear thermal expansion depends on the material, so investigating the coefficient provides a hint about what material the bar is. Solve $α$ :

$\begin{aligned} l & = l_{0} + l_{0} α Δ T & | | - l_{0} \\ l - l_{0} & = l_{0} α Δ T & | | : l_{0} Δ T \\ α & = \frac{l - l_{0}}{l_{0} Δ T} \end{aligned}$

Insert values:

$l_{0} = 826.0 mm$
$l = 830.7 mm$
$Δ T = 269 °C - 23 °C = 246 °C$

We get

$\begin{aligned} α & = \frac{830.7 mm - 826.0 mm}{826.0 mm \cdot 246 °C} \\ = 0.000 023 13 \dots \frac{1}{^{\circ} C} \approx 23.1 \cdot 10^{- 6} \frac{1}{^{\circ} C} \end{aligned}$

Aluminium has a coefficient of linear thermal expansion of $23.1 \cdot 10^{- 6} \frac{1}{^{\circ} C}$ , so the material is probably aluminium.

Based on its thermal expansion coefficient, the bar is likely made of aluminium.

Lisää aiheesta

Practical study – build a thermometer

In small groups, build a thermometer that is based on changes in the height of a column of liquid. You will need a liquid that has good thermal expansion (such as ethanol), a container for the liquid, and a narrow, transparent tube that can be attached to the container. Measure the height of the column of liquid at different temperatures and create a temperature scale on the tube.

Lisää aiheesta

Pause and reflect

1. Which expands more as the temperature increases?

steel
glass
Both expand equally.

2. The change in length Δl in thermal expansion is modelled using the formula

$Δ l = α Δ T$
$Δ l = α l_{0} Δ T$
$Δ l = α l_{0} T$
$Δ l = α T$

3. The width of both the pane of glass and the steel frame (0.25% carbon) is 2314 mm. The temperature rises from –15°C to 25°C. In this case, the change in temperature ΔT in kelvins is K.

4. How much does the glass expand by? Give your answer to the nearest tenth of a millimetre. mm.

5. How much does the steel frame expand by? Give your answer to the nearest tenth of a millimetre. mm.

1. Absolute zero is

–273.15 K.
0 °C.
0 K.
273.15 °C.

Dry ice rapidly changes into a gas at room temperature.

2. Carbon dioxide (CO₂) sublimates at –79°C. In kelvins, this is approximately

57 K.
180 K.
216 K.
330 K.

The Sun’s warmth makes water evaporate and causes clouds to form.

3. The temperature of the surface of the Sun is 5778 K. In degrees Celsius, this is approximately

5 505 °C.
5 778 °C.
5 878 °C.
6 051 °C.

A weather station at Verkhoyansk in Yakutia, Russia.

The greatest change in temperature worldwide was recorded in Verkhoyansk, Russia, where the lowest winter temperature was –68°C and the highest summer temperature was 37°C.

4. The change in temperature between these two extreme temperatures in degrees Celsius is °C.

5. The difference between extreme temperatures in Verkhoyansk in kelvins is K.

Lisää aiheesta

Palvelun tarjoaa e-Oppi Oy ja Star Cloud OÜ

Rekisteröidy

Edistyminen

	Tehtävät
	Luku on suoritettu

Measuring temperatures and thermal expansion

Key questions

Lisää aiheesta

Temperature as a quantity

Lisää aiheesta

Measuring temperature

Different kinds of thermometers

Lisää aiheesta

Linear thermal expansion

Measurement results

Linear thermal expansion

Lisää aiheesta

Thermal expansion in nature

Lisää aiheesta

Volumetric thermal expansion

Volumetric thermal expansion

Lisää aiheesta

Examples

Example 1

Solution to example 1

Solution to section a)

Solution to section b)

Example 2

Solution to example 2

Example 3

Solution to example 3

Solution to section a)

Solution to section b)

Example 4

Solution to example 4

Lisää aiheesta

Practical study – build a thermometer

Lisää aiheesta

Pause and reflect

Lisää aiheesta

Lisää materiaali

Lisättävät tiedostot

Ilmoita virheestä

Ilmoita tänne vain alustaan ja sisältöön liittyvistä virheistä. Pyydämme kuvailemaan virhettä mahdollisimman tarkasti.

Liitä halutessasi mukaan yhteystietosi (sähköpostiosoite, puhelin).

Tee rasti ruutuun, näin autat meitä taistelussa roskapostia vastaan

Opiq käyttää evästeitä